Southern California Analysis & PDE (SCAPDE) meeting
Nov 13-14, 2010, UCLA
Location: Mathematical Sciences building, MS 6627
Schedule:
Saturday, Nov 13
- 8:00-9:00 Breakfast
- 9:00-10:00 Scott Armstrong (Chicago) Random homogenization of a neutron multigroup diffusion model
- I will present some recent results obtained in joint work with Souganidis. We study an spectral problem for an elliptic system which arises in the theory of nuclear reactors. The model has been studied in the periodic setting in recent years by Allaire, Capdeboscq, and others. To obtain results for random (stationary ergodic) media we need new ideas to deal with the usual "lack of compactness". Even in the i.i.d. case we find behavior not seen in the periodic case.
- 10:30-11:30 Benedek Valko (Madison) Interpolation of Gibbs measures with White Noise for Hamiltonian PDE
- We consider Gibbs measures corresponding to certain Hamiltonian PDEs (KdV, mKdV and cubic NLS) on the circle. I will describe a way one can interpolate between these measures and the mean zero white noise so that the interpolating measures converge weakly to the white noise.
As an application one obtains (another) proof for the invariance of white noise for KdV on the circle.
(joint with T. Oh and J. Quastel)
- 11:30-1:00 Lunch
- 1:00-2:00 Magda Czubak (Toronto): Non-uniqueness of the Navier-Stokes equation in the hyperbolic setting
- We consider the Navier-Stokes equation on the two dimensional hyperbolic space with constant sectional curvature $−a^2$. We prove non-uniqueness of smooth solutions which have finite energy, finite dissipation, and satisfy the global energy inequality. We also obtain a corresponding result on a more general negatively curved manifold for a modified geometric version of the Navier-Stokes equation. Finally, as a corollary we show a lack of the Liouville theorem in the hyperbolic setting both in two and three dimensions. This is joint work with Chi Hin Chan.
- 2:30-3:30 Andrea Nahmod (U. Mass.): Periodic derivative NLS: almost sure global existence and invariance of its weighted Wiener measure
- In the past few years, methods such as those by J. Bourgain (high-low method) on the one hand and by J. Colliander, M. Keel, G. Staffilani, H. Takaoka and T. Tao (I-method or method of almost conservation laws) on the other, have been applied to study the global in time existence of dispersive equations at regularities which are right below or in between those corresponding to conserved quantities. As it turns out however, for many dispersive equations and systems there still remains a gap between the local in time results and those that could be globally achieved. In those cases, it seems natural to return to one of Bourgain's early approaches for periodic dispersive equations where global in time existence was studied in the almost sure sense via the existence and invariance of the associated Gibbs measure (following the works of Lebowitz, Rose and Speer and Zhidkov). Failure to show global existence by Bourgain's high-low method or the I-method might come from certain `exceptional' initial data set, and the virtue of the Gibbs measure is that it does not see that exceptional set. At the same time, the invariance of the Gibbs measure, just like the usual conserved quantities, can be used to control the growth in time of those solutions in its support and extend the local in time solutions to global ones almost surely.
The difficulty in this approach lies in the actual construction of the associated Gibbs measure(or weighted Wiener measure) and in showing both its invariance under the flow and the almost sure global well-posedness.
In this talk we apply this approach to the 1D periodic derivative nonlinear Schrodinger equation.
- 3:30-4:00 Tea
- 4:00-5:00 Thomas Chen (UT Austin): Mean field limits for interacting Bose gases and the Cauchy problem for Gross-Pitaevskii hierarchies
- This talk surveys some recent results, all based on joint work with Natasa Pavlovic, related to the dynamics of Bose gases, and the Cauchy problem for Gross-Pitaevskii (GP) hierarchies. A GP hierarchy is an infinite system of coupled partial differential equations describing an interacting Bose gas in a mean field limit. First, we describe how the quintic nonlinear Schrodinger equation is derived from an N-body Schrodinger system with 3-body interactions and an associated GP hierarchy. Then, the local well-posedness theory for more general GP hierarchies is addressed, for focusing, defocusing, cubic and quintic interactions. In particular, the occurrence of blowup solutions is discussed (joint work with N. Pavlovic and N. Tzirakis). Furthermore, we present new conserved energy functionals which we apply to give a new proof of uniqueness of solutions of GP hierarchies, and to extend local to global well-posedness.
There will be informal groups going out to dinner on Saturday evening (see below).
Sunday, Nov 14
- 8:00-8:30 Breakfast
- 8:30-9:30 David Damanik (Rice): The Square Fibonacci Hamiltonian and Arithmetic Sums of Cantor Sets
- Motivated by numerical observations of Even-Dar Mandel and Lifshitz we investigate the structure of the spectrum of the Square Fibonacci Hamiltonian and confirm their observations in the small coupling regime, where we show the absence of gaps in the spectrum, as well as in the large coupling regime, where the spectrum turns out to be nowhere dense. The open problems in the intermediate coupling regime will be briefly discussed. The talk is based on joint work with Anton Gorodetski.
- 9:45-10:45 Juhi Jang (UC Riverside): Well-posedness theory for compressible Euler equations in a physical vacuum
- An interesting problem in gas and fluid dynamics is to understand the behavior of vacuum states, namely the behavior of the system in the presence of vacuum. A particular interest is so called physical vacuum which naturally arises in physical problems. The main difficulty lies in the fact that the physical systems become degenerate along the boundary. I'll present the well-posedness result of 3D compressible Euler equations for polytropic gases in the presence of physical vacuum based on a hyperbolic type of energy estimates which consist in the instant energy estimates and the curl estimates. This is a joint work with Nader Masmoudi.
- 11:15-12:15 Alex Ionescu (Princeton): On the uniqueness of stationary black holes in vacuum
- I will discuss the uniqueness problem of the Kerr solution among regular, stationary solutions of the Einstein vacuum equation. Then I will discuss some recent joint work with S. Klainerman and S. Alexakis of this problem.
There will be an informal (free) registration during the conference.
A list of hotels near UCLA can be found here. A campus map can be found here.
Thanks to NSF grant DMS-0649473, we now have some funds to support graduate student and postdoctoral travel to attend this conference. Please contact tao@math.ucla.edu for details on this (or for any other inquiries). Reimbursement forms will be provided at the conference.
Navigation
To get to campus, consult this Google map, centered on the Westwood Blvd parking booth.
Here is a map to help with on-campus navigaion:
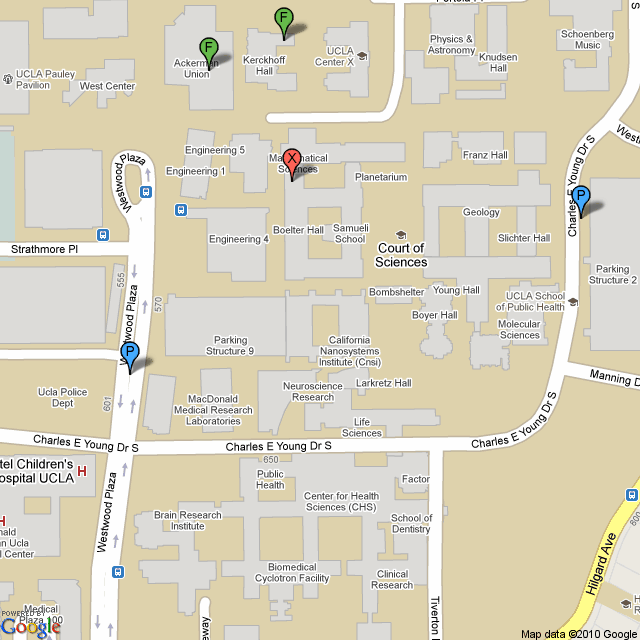
Key:
X = Location of lectures: Math Sciences building, room 6627.
P = Parking: (1) Parking booth on Westwood Plaza (=Blvd). (2) Self-service parking entrance to structure #2.
F = On-campus food: Kerckhoff coffee house (salads/sandwiches) and Ackerman Union (Sbarro/Panda Express/Carls Jr./Rubio's)
Tiverton house is here on the east side Tiverton (almost) at the intersection with Le Conte, just off the bottom of the map.
Dining
There are numerous eateries in Westwood village and further down Westwood Blvd. Here is a selection:
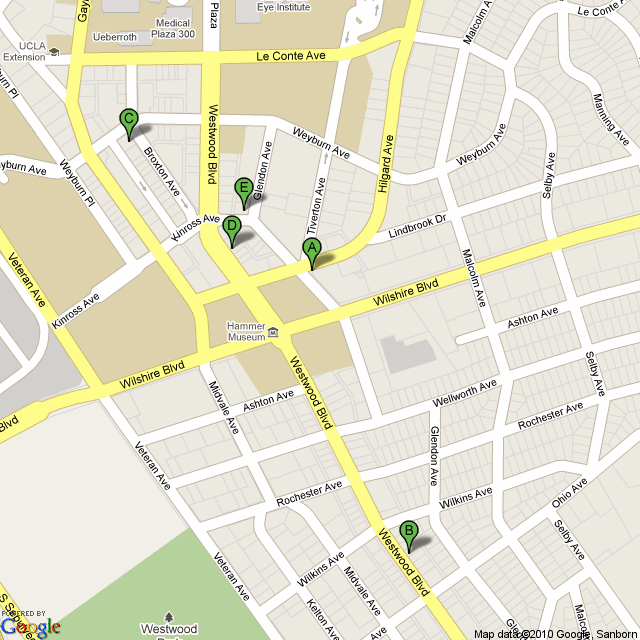
A = Palomino Very nice, but a little pricey.
B = Flame Persian Cuisine One of several Persian restaurants; this neighbourhood is known as "Little Persia".
C = California Pizza Kitchen Pizza.
D = Noodle Planet Very economical.
E = Westwood Brewing Co It's a bar.
Local organisers: John Garnett, Rowan Killip, Terence Tao, Christoph Thiele, Monica Visan
Global organisers: Hans Lindblad, Jacob Sterbenz
Previous SCAPDE meetings:
- Winter 2010, UC San Diego
- Fall 2009, UC Irvine
- Winter 2009, UCLA
- Winter 2008, UC San Diego
- Fall 2002, UCLA
- Spring 2000,UC San Diego


